Long-term correlation

If a signal is truly random we will never observe any long term correlation (i.e., no power concentration in the low frequency region). However, some operations can create such correlations. Obviously the simplest one is to add such a structure to the random signal, which is not currently what we are inspecting. A bad quantization method can also create correlations in the low frequency region in a random signal. In this case an artificial DC power is added to our signal and that creates a correlation in the low frequency region. In this case one can say that: ``Correlation is in the eye of the beholder''; meaning that it is a correlation in the process of our measurement and not in the signal itself. 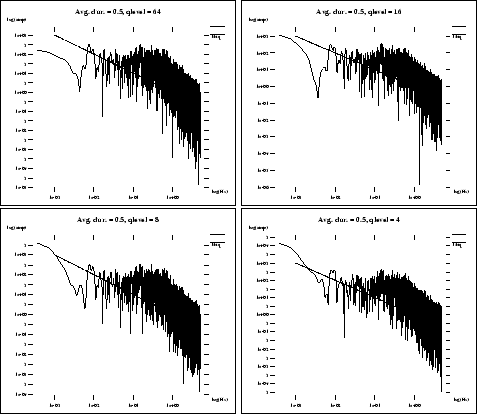Figure 4-5 shows the effect of truncating values for quantization rather than rounding them to the nearest integer value. Notice that as the number of quantization levels gets smaller the low frequency power gets larger.

Figure 4-5: In this figure the effect of bad quantization of a random signal is illustrated. The values of a random signal are truncated to the quantized level rather than being rounded to the nearest level. This figures should be compared to the first illustration of figure 4-4. Notice how the low frequency power increases as we use fewer quantization levels.

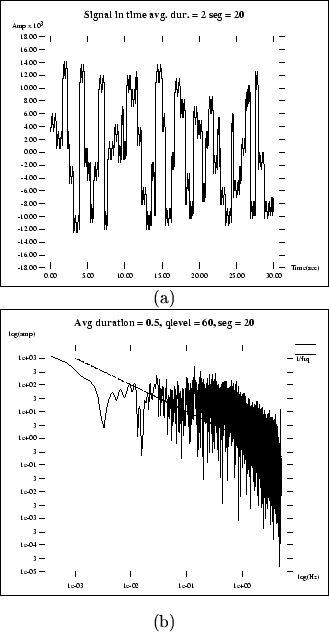
One other way to create low frequency power is to add deterministic structures on top of the random values in micro-structures. Figure 4-6 shows the power spectrum and the first 30 seconds of a random signal with average note duration of .5 seconds with a simple vibrato added to every note. The vibrato's period is determined by the duration of the note.

Figure 4-6: The first 30 seconds and the power spectrum of a random signal with a simple deterministic shape added to it is illustrated. The deterministic shaped is scaled to the duration of the note. Notice that such a process shows up as low frequency (i.e. long term correlation) on the power spectrum.